One of the elements of primitive level algebra is the logarithm. The name comes from Greek language from the word “number” or “power” and means the power to which the number in the base must be raised to find the final number.
Types of logarithms
- log a b – logarithm of the number b to base a (a > 0, a ≠ 1, b > 0);
- log b – decimal logarithm (logarithm to base 10, a = 10);
- ln b – natural logarithm (logarithm to base e, a = e).
How to solve logarithms?
The logarithm of b to base a is an exponent that requires b to be raised to base a. The result obtained is pronounced like this: “logarithm of b to base a.” The solution to logarithmic problems is that you need to determine the given power in numbers from the specified numbers. There are some basic rules to determine or solve the logarithm, as well as convert the notation itself. Using them, the solution is made logarithmic equations, derivatives are found, integrals are solved, and many other operations are performed. Basically, the solution to the logarithm itself is its simplified notation. Below are the basic formulas and properties:
For any a ; a > 0; a ≠ 1 and for any x ; y > 0.
- a log a b = b – basic logarithmic identity
- log a 1 = 0
- loga a = 1
- log a (x y) = log a x + log a y
- log a x/ y = log a x – log a y
- log a 1/x = -log a x
- log a x p = p log a x
- log a k x = 1/k log a x , for k ≠ 0
- log a x = log a c x c
- log a x = log b x/ log b a – formula for moving to a new base
- log a x = 1/log x a
How to solve logarithms - step-by-step instructions for solving
- First, write down the required equation.
Please note: if the base logarithm is 10, then the entry is shortened, resulting in a decimal logarithm. If it's worth natural number e, then we write it down, reducing it to the natural logarithm. This means that the result of all logarithms is the power to which the base number is raised to obtain the number b.
Directly, the solution lies in calculating this degree. Before solving an expression with a logarithm, it must be simplified according to the rule, that is, using formulas. You can find the main identities by going back a little in the article.
Adding and subtracting logarithms with two different numbers, but with the same bases, replace with one logarithm with the product or division of the numbers b and c, respectively. In this case, you can apply the formula for moving to another base (see above).
If you use expressions to simplify a logarithm, there are some limitations to consider. And that is: the base of the logarithm a is only a positive number, but not equal to one. The number b, like a, must be greater than zero.
There are cases where, by simplifying an expression, you will not be able to calculate the logarithm numerically. It happens that such an expression does not make sense, because many powers are irrational numbers. Under this condition, leave the power of the number as a logarithm.
main properties.
- logax + logay = loga(x y);
- logax − logay = loga (x: y).
identical grounds
Log6 4 + log6 9.
Now let's complicate the task a little.
Examples of solving logarithms
What if the base or argument of a logarithm is a power? Then the exponent of this degree can be taken out of the sign of the logarithm according to the following rules:
Of course, all these rules make sense if the ODZ of the logarithm is observed: a > 0, a ≠ 1, x >
Task. Find the meaning of the expression:
Transition to a new foundation
Let the logarithm logax be given. Then for any number c such that c > 0 and c ≠ 1, the equality is true:
Task. Find the meaning of the expression:
See also:
Basic properties of the logarithm
1.
2.
3.
4.
5. 
6.
7.
8.
9.
10.
11. 
12.
13.
14. 
15.
The exponent is 2.718281828…. To remember the exponent, you can study the rule: the exponent is equal to 2.7 and twice the year of birth of Leo Nikolaevich Tolstoy.
Basic properties of logarithms
Knowing this rule, you will know and exact value exhibitors, and the date of birth of Leo Tolstoy.
Examples for logarithms
Logarithm expressions
Example 1.
A). x=10ac^2 (a>0,c>0).
Using properties 3.5 we calculate 
2.
3. 
4. 
Where 
.
Example 2. Find x if
Example 3. Let the value of logarithms be given
Calculate log(x) if
Basic properties of logarithms
Logarithms, like any numbers, can be added, subtracted and transformed in every way. But since logarithms are not exactly ordinary numbers, there are rules here, which are called main properties.
You definitely need to know these rules - not a single serious logarithmic problem can be solved without them. In addition, there are very few of them - you can learn everything in one day. So let's get started.
Adding and subtracting logarithms
Consider two logarithms with the same bases: logax and logay. Then they can be added and subtracted, and:
- logax + logay = loga(x y);
- logax − logay = loga (x: y).
So, the sum of logarithms is equal to the logarithm of the product, and the difference is equal to the logarithm of the quotient. Please note: the key point here is identical grounds. If the reasons are different, these rules do not work!
These formulas will help you calculate logarithmic expression even when its individual parts are not counted (see the lesson “What is a logarithm”). Take a look at the examples and see:
Since logarithms have the same bases, we use the sum formula:
log6 4 + log6 9 = log6 (4 9) = log6 36 = 2.
Task. Find the value of the expression: log2 48 − log2 3.
The bases are the same, we use the difference formula:
log2 48 − log2 3 = log2 (48: 3) = log2 16 = 4.
Task. Find the value of the expression: log3 135 − log3 5.
Again the bases are the same, so we have:
log3 135 − log3 5 = log3 (135: 5) = log3 27 = 3.
As you can see, the original expressions are made up of “bad” logarithms, which are not calculated separately. But after the transformations, completely normal numbers are obtained. Many are built on this fact tests. Yes, test-like expressions are offered in all seriousness (sometimes with virtually no changes) on the Unified State Examination.
Extracting the exponent from the logarithm
It is easy to see that the last rule follows the first two. But it’s better to remember it anyway - in some cases it will significantly reduce the amount of calculations.
Of course, all these rules make sense if the ODZ of the logarithm is observed: a > 0, a ≠ 1, x > 0. And one more thing: learn to apply all formulas not only from left to right, but also vice versa, i.e. You can enter the numbers before the logarithm sign into the logarithm itself. This is what is most often required.
Task. Find the value of the expression: log7 496.
Let's get rid of the degree in the argument using the first formula:
log7 496 = 6 log7 49 = 6 2 = 12
Task. Find the meaning of the expression:
Note that the denominator contains a logarithm, the base and argument of which are exact powers: 16 = 24; 49 = 72. We have:
I think the last example requires some clarification. Where have logarithms gone? Until the very last moment we work only with the denominator.
Logarithm formulas. Logarithms examples solutions.
We presented the base and argument of the logarithm standing there in the form of powers and took out the exponents - we got a “three-story” fraction.
Now let's look at the main fraction. The numerator and denominator contain the same number: log2 7. Since log2 7 ≠ 0, we can reduce the fraction - 2/4 will remain in the denominator. According to the rules of arithmetic, the four can be transferred to the numerator, which is what was done. The result was the answer: 2.
Transition to a new foundation
Speaking about the rules for adding and subtracting logarithms, I specifically emphasized that they only work with the same bases. What if the reasons are different? What if they are not exact powers of the same number?
Formulas for transition to a new foundation come to the rescue. Let us formulate them in the form of a theorem:
Let the logarithm logax be given. Then for any number c such that c > 0 and c ≠ 1, the equality is true:
In particular, if we set c = x, we get:
From the second formula it follows that the base and argument of the logarithm can be swapped, but in this case the entire expression is “turned over”, i.e. the logarithm appears in the denominator.
These formulas are rarely found in conventional numerical expressions. It is possible to evaluate how convenient they are only when solving logarithmic equations and inequalities.
However, there are problems that cannot be solved at all except by moving to a new foundation. Let's look at a couple of these:
Task. Find the value of the expression: log5 16 log2 25.
Note that the arguments of both logarithms contain exact powers. Let's take out the indicators: log5 16 = log5 24 = 4log5 2; log2 25 = log2 52 = 2log2 5;
Now let’s “reverse” the second logarithm:
Since the product does not change when rearranging factors, we calmly multiplied four and two, and then dealt with logarithms.
Task. Find the value of the expression: log9 100 lg 3.
The base and argument of the first logarithm are exact powers. Let's write this down and get rid of the indicators:
Now let's get rid of the decimal logarithm by moving to a new base:
Basic logarithmic identity
Often in the solution process it is necessary to represent a number as a logarithm to a given base. In this case, the following formulas will help us:
In the first case, the number n becomes the exponent in the argument. The number n can be absolutely anything, because it is just a logarithm value.
The second formula is actually a paraphrased definition. That's what it's called: .
In fact, what happens if the number b is raised to such a power that the number b to this power gives the number a? That's right: the result is the same number a. Read this paragraph carefully again - many people get stuck on it.
Like formulas for moving to a new base, the basic logarithmic identity is sometimes the only possible solution.
Task. Find the meaning of the expression:
Note that log25 64 = log5 8 - simply took the square from the base and argument of the logarithm. Taking into account the rules for multiplying powers with the same base, we get:
If anyone doesn’t know, this was a real task from the Unified State Exam :)
Logarithmic unit and logarithmic zero
In conclusion, I will give two identities that can hardly be called properties - rather, they are consequences of the definition of the logarithm. They constantly appear in problems and, surprisingly, create problems even for “advanced” students.
- logaa = 1 is. Remember once and for all: the logarithm to any base a of that base itself is equal to one.
- loga 1 = 0 is. The base a can be anything, but if the argument contains one, the logarithm is equal to zero! Because a0 = 1 is a direct consequence of the definition.
That's all the properties. Be sure to practice putting them into practice! Download the cheat sheet at the beginning of the lesson, print it out, and solve the problems.
See also:
The logarithm of b to base a denotes the expression. To calculate the logarithm means to find a power x () at which the equality is satisfied
Basic properties of the logarithm
It is necessary to know the above properties, since almost all problems and examples related to logarithms are solved on their basis. The rest of the exotic properties can be derived through mathematical manipulations with these formulas
1.
2.
3.
4.
5.
6.
7.
8.
9.
10.
11.
12.
13.
14.
15.
When calculating the formula for the sum and difference of logarithms (3.4) you come across quite often. The rest are somewhat complex, but in a number of tasks they are indispensable for simplifying complex expressions and calculating their values.
Common cases of logarithms
Some of the most common logarithms are those in which the base is equal to ten, exponential or two.
The logarithm to base ten is usually called the decimal logarithm and is simply denoted by lg(x).
It is clear from the recording that the basics are not written in the recording. For example
Natural logarithm is a logarithm with an exponent as its basis (denoted by ln(x)).
The exponent is 2.718281828…. To remember the exponent, you can study the rule: the exponent is equal to 2.7 and twice the year of birth of Leo Nikolaevich Tolstoy. Knowing this rule, you will know both the exact value of the exponent and the date of birth of Leo Tolstoy.
And another important logarithm to base two is denoted by
The derivative of the logarithm of a function is equal to one divided by the variable
The integral or antiderivative logarithm is determined by the relationship
The given material is enough for you to solve a wide class of problems related to logarithms and logarithms. To help you understand the material, I will give just a few common examples from school curriculum and universities.
Examples for logarithms
Logarithm expressions
Example 1.
A). x=10ac^2 (a>0,c>0).
Using properties 3.5 we calculate
2.
By the property of difference of logarithms we have
3.
Using properties 3.5 we find
4. Where .
A seemingly complex expression is simplified to form using a number of rules
Finding logarithm values
Example 2. Find x if
Solution. For calculation, we apply to the last term 5 and 13 properties
We put it on record and mourn
Since the bases are equal, we equate the expressions
Logarithms. Entry level.
Let the value of logarithms be given
Calculate log(x) if
Solution: Let's take a logarithm of the variable to write the logarithm through the sum of its terms
This is just the beginning of our acquaintance with logarithms and their properties. Practice calculations, enrich your practical skills - you will soon need the knowledge you gain to solve logarithmic equations. Having studied the basic methods for solving such equations, we will expand your knowledge to another equally important topic - logarithmic inequalities...
Basic properties of logarithms
Logarithms, like any numbers, can be added, subtracted and transformed in every way. But since logarithms are not exactly ordinary numbers, there are rules here, which are called main properties.
You definitely need to know these rules - not a single serious logarithmic problem can be solved without them. In addition, there are very few of them - you can learn everything in one day. So let's get started.
Adding and subtracting logarithms
Consider two logarithms with the same bases: logax and logay. Then they can be added and subtracted, and:
- logax + logay = loga(x y);
- logax − logay = loga (x: y).
So, the sum of logarithms is equal to the logarithm of the product, and the difference is equal to the logarithm of the quotient. Please note: the key point here is identical grounds. If the reasons are different, these rules do not work!
These formulas will help you calculate a logarithmic expression even when its individual parts are not considered (see the lesson “What is a logarithm”). Take a look at the examples and see:
Task. Find the value of the expression: log6 4 + log6 9.
Since logarithms have the same bases, we use the sum formula:
log6 4 + log6 9 = log6 (4 9) = log6 36 = 2.
Task. Find the value of the expression: log2 48 − log2 3.
The bases are the same, we use the difference formula:
log2 48 − log2 3 = log2 (48: 3) = log2 16 = 4.
Task. Find the value of the expression: log3 135 − log3 5.
Again the bases are the same, so we have:
log3 135 − log3 5 = log3 (135: 5) = log3 27 = 3.
As you can see, the original expressions are made up of “bad” logarithms, which are not calculated separately. But after the transformations, completely normal numbers are obtained. Many tests are based on this fact. Yes, test-like expressions are offered in all seriousness (sometimes with virtually no changes) on the Unified State Examination.
Extracting the exponent from the logarithm
Now let's complicate the task a little. What if the base or argument of a logarithm is a power? Then the exponent of this degree can be taken out of the sign of the logarithm according to the following rules:
It is easy to see that the last rule follows the first two. But it’s better to remember it anyway - in some cases it will significantly reduce the amount of calculations.
Of course, all these rules make sense if the ODZ of the logarithm is observed: a > 0, a ≠ 1, x > 0. And one more thing: learn to apply all formulas not only from left to right, but also vice versa, i.e. You can enter the numbers before the logarithm sign into the logarithm itself.
How to solve logarithms
This is what is most often required.
Task. Find the value of the expression: log7 496.
Let's get rid of the degree in the argument using the first formula:
log7 496 = 6 log7 49 = 6 2 = 12
Task. Find the meaning of the expression:
Note that the denominator contains a logarithm, the base and argument of which are exact powers: 16 = 24; 49 = 72. We have:
I think the last example requires some clarification. Where have logarithms gone? Until the very last moment we work only with the denominator. We presented the base and argument of the logarithm standing there in the form of powers and took out the exponents - we got a “three-story” fraction.
Now let's look at the main fraction. The numerator and denominator contain the same number: log2 7. Since log2 7 ≠ 0, we can reduce the fraction - 2/4 will remain in the denominator. According to the rules of arithmetic, the four can be transferred to the numerator, which is what was done. The result was the answer: 2.
Transition to a new foundation
Speaking about the rules for adding and subtracting logarithms, I specifically emphasized that they only work with the same bases. What if the reasons are different? What if they are not exact powers of the same number?
Formulas for transition to a new foundation come to the rescue. Let us formulate them in the form of a theorem:
Let the logarithm logax be given. Then for any number c such that c > 0 and c ≠ 1, the equality is true:
In particular, if we set c = x, we get:
From the second formula it follows that the base and argument of the logarithm can be swapped, but in this case the entire expression is “turned over”, i.e. the logarithm appears in the denominator.
These formulas are rarely found in ordinary numerical expressions. It is possible to evaluate how convenient they are only when solving logarithmic equations and inequalities.
However, there are problems that cannot be solved at all except by moving to a new foundation. Let's look at a couple of these:
Task. Find the value of the expression: log5 16 log2 25.
Note that the arguments of both logarithms contain exact powers. Let's take out the indicators: log5 16 = log5 24 = 4log5 2; log2 25 = log2 52 = 2log2 5;
Now let’s “reverse” the second logarithm:
Since the product does not change when rearranging factors, we calmly multiplied four and two, and then dealt with logarithms.
Task. Find the value of the expression: log9 100 lg 3.
The base and argument of the first logarithm are exact powers. Let's write this down and get rid of the indicators:
Now let's get rid of the decimal logarithm by moving to a new base:
Basic logarithmic identity
Often in the solution process it is necessary to represent a number as a logarithm to a given base. In this case, the following formulas will help us:
In the first case, the number n becomes the exponent in the argument. The number n can be absolutely anything, because it is just a logarithm value.
The second formula is actually a paraphrased definition. That's what it's called: .
In fact, what happens if the number b is raised to such a power that the number b to this power gives the number a? That's right: the result is the same number a. Read this paragraph carefully again - many people get stuck on it.
Like formulas for moving to a new base, the basic logarithmic identity is sometimes the only possible solution.
Task. Find the meaning of the expression:
Note that log25 64 = log5 8 - simply took the square from the base and argument of the logarithm. Taking into account the rules for multiplying powers with the same base, we get:
If anyone doesn’t know, this was a real task from the Unified State Exam :)
Logarithmic unit and logarithmic zero
In conclusion, I will give two identities that can hardly be called properties - rather, they are consequences of the definition of the logarithm. They constantly appear in problems and, surprisingly, create problems even for “advanced” students.
- logaa = 1 is. Remember once and for all: the logarithm to any base a of that base itself is equal to one.
- loga 1 = 0 is. The base a can be anything, but if the argument contains one, the logarithm is equal to zero! Because a0 = 1 is a direct consequence of the definition.
That's all the properties. Be sure to practice putting them into practice! Download the cheat sheet at the beginning of the lesson, print it out, and solve the problems.
The basic properties of the natural logarithm, graph, domain of definition, set of values, basic formulas, derivative, integral, expansion in power series and representation of the function ln x using complex numbers.
Definition
Natural logarithm is the function y = ln x, the inverse of the exponential, x = e y, and is the logarithm to the base of the number e: ln x = log e x.
The natural logarithm is widely used in mathematics because its derivative has the simplest form: (ln x)′ = 1/ x.
Based on definitions, the base of the natural logarithm is the number e:
e ≅ 2.718281828459045...;
.
Graph of the function y = ln x.
Graph of natural logarithm (functions y = ln x) is obtained from the exponential graph mirror image relative to the straight line y = x.
The natural logarithm is defined for positive values of the variable x. It increases monotonically in its domain of definition.
At x → 0 the limit of the natural logarithm is minus infinity (-∞).
As x → + ∞, the limit of the natural logarithm is plus infinity (+ ∞). For large x, the logarithm increases quite slowly. Any power function x a with a positive exponent a grows faster than the logarithm.
Properties of the natural logarithm
Domain of definition, set of values, extrema, increase, decrease
The natural logarithm is a monotonically increasing function, so it has no extrema. The main properties of the natural logarithm are presented in the table.
ln x values
ln 1 = 0
Basic formulas for natural logarithms
Formulas following from the definition of the inverse function:
The main property of logarithms and its consequences
Base replacement formula
Any logarithm can be expressed in terms of natural logarithms using the base substitution formula:
Proofs of these formulas are presented in the section "Logarithm".
Inverse function
The inverse of the natural logarithm is the exponent.
If , then
If, then.
Derivative ln x
Derivative of the natural logarithm:
.
Derivative of the natural logarithm of modulus x:
.
Derivative of nth order:
.
Deriving formulas > > >
Integral
The integral is calculated by integration by parts:
.
So,
Expressions using complex numbers
Consider the function of the complex variable z:
.
Let's express the complex variable z via module r and argument φ
:
.
Using the properties of the logarithm, we have:
.
Or
.
The argument φ is not uniquely defined. If you put
, where n is an integer,
it will be the same number for different n.
Therefore, the natural logarithm, as a function of a complex variable, is not a single-valued function.
Power series expansion
When the expansion takes place:
Used literature:
I.N. Bronstein, K.A. Semendyaev, Handbook of mathematics for engineers and college students, “Lan”, 2009.
\(a^(b)=c\) \(\Leftrightarrow\) \(\log_(a)(c)=b\)
Let's explain it more simply. For example, \(\log_(2)(8)\) is equal to the power to which \(2\) must be raised to get \(8\). From this it is clear that \(\log_(2)(8)=3\).
|
Examples: |
\(\log_(5)(25)=2\) |
because \(5^(2)=25\) |
||
|
\(\log_(3)(81)=4\) |
because \(3^(4)=81\) |
|||
|
\(\log_(2)\)\(\frac(1)(32)\) \(=-5\) |
because \(2^(-5)=\)\(\frac(1)(32)\) |
Argument and base of logarithm
Any logarithm has the following “anatomy”:
The argument of a logarithm is usually written at its level, and the base is written in subscript closer to the logarithm sign. And this entry reads like this: “logarithm of twenty-five to base five.”
How to calculate logarithm?
To calculate the logarithm, you need to answer the question: to what power should the base be raised to get the argument?
For example, calculate the logarithm: a) \(\log_(4)(16)\) b) \(\log_(3)\)\(\frac(1)(3)\) c) \(\log_(\sqrt (5))(1)\) d) \(\log_(\sqrt(7))(\sqrt(7))\) e) \(\log_(3)(\sqrt(3))\)
a) To what power must \(4\) be raised to get \(16\)? Obviously the second one. That's why:
\(\log_(4)(16)=2\)
\(\log_(3)\)\(\frac(1)(3)\) \(=-1\)
c) To what power must \(\sqrt(5)\) be raised to get \(1\)? What power makes any number one? Zero, of course!
\(\log_(\sqrt(5))(1)=0\)
d) To what power must \(\sqrt(7)\) be raised to obtain \(\sqrt(7)\)? Firstly, any number to the first power is equal to itself.
\(\log_(\sqrt(7))(\sqrt(7))=1\)
e) To what power must \(3\) be raised to obtain \(\sqrt(3)\)? From we know that is a fractional power, which means square root is the power of \(\frac(1)(2)\) .
\(\log_(3)(\sqrt(3))=\)\(\frac(1)(2)\)
Example : Calculate logarithm \(\log_(4\sqrt(2))(8)\)
Solution :
|
\(\log_(4\sqrt(2))(8)=x\) |
We need to find the value of the logarithm, let's denote it as x. Now let's use the definition of a logarithm: |
|
|
\((4\sqrt(2))^(x)=8\) |
What connects \(4\sqrt(2)\) and \(8\)? Two, because both numbers can be represented by twos: |
|
|
\(((2^(2)\cdot2^(\frac(1)(2))))^(x)=2^(3)\) |
On the left we use the properties of the degree: \(a^(m)\cdot a^(n)=a^(m+n)\) and \((a^(m))^(n)=a^(m\cdot n)\) |
|
|
\(2^(\frac(5)(2)x)=2^(3)\) |
The bases are equal, we move on to equality of indicators |
|
|
\(\frac(5x)(2)\) \(=3\) |
|
Multiply both sides of the equation by \(\frac(2)(5)\) |
|
|
The resulting root is the value of the logarithm |
Answer : \(\log_(4\sqrt(2))(8)=1,2\)
Why was the logarithm invented?
To understand this, let's solve the equation: \(3^(x)=9\). Just match \(x\) to make the equality work. Of course, \(x=2\).
Now solve the equation: \(3^(x)=8\).What is x equal to? That's the point.
The smartest ones will say: “X is a little less than two.” How exactly to write this number? To answer this question, the logarithm was invented. Thanks to him, the answer here can be written as \(x=\log_(3)(8)\).
I want to emphasize that \(\log_(3)(8)\), like any logarithm is just a number. Yes, it looks unusual, but it’s short. Because if we wanted to write it as a decimal, it would look like this: \(1.892789260714.....\)
Example : Solve the equation \(4^(5x-4)=10\)
Solution :
|
\(4^(5x-4)=10\) |
\(4^(5x-4)\) and \(10\) cannot be brought to the same base. This means you can’t do without a logarithm. Let's use the definition of logarithm: |
|
|
\(\log_(4)(10)=5x-4\) |
Let's flip the equation so that X is on the left |
|
|
\(5x-4=\log_(4)(10)\) |
Before us. Let's move \(4\) to the right. And don't be afraid of the logarithm, treat it like an ordinary number. |
|
|
\(5x=\log_(4)(10)+4\) |
Divide the equation by 5 |
|
|
\(x=\)\(\frac(\log_(4)(10)+4)(5)\) |
|
This is our root. Yes, it looks unusual, but they don’t choose the answer. |
Answer : \(\frac(\log_(4)(10)+4)(5)\)
Decimal and natural logarithms
As stated in the definition of a logarithm, its base can be any positive number except one \((a>0, a\neq1)\). And among all the possible bases, there are two that occur so often that a special short notation was invented for logarithms with them:
Natural logarithm: a logarithm whose base is Euler's number \(e\) (equal to approximately \(2.7182818…\)), and the logarithm is written as \(\ln(a)\).
That is, \(\ln(a)\) is the same as \(\log_(e)(a)\)
Decimal Logarithm: A logarithm whose base is 10 is written \(\lg(a)\).
That is, \(\lg(a)\) is the same as \(\log_(10)(a)\), where \(a\) is some number.
Basic logarithmic identity
Logarithms have many properties. One of them is called the “Basic Logarithmic Identity” and looks like this:
| \(a^(\log_(a)(c))=c\) |
This property follows directly from the definition. Let's see exactly how this formula came about.
Let us recall a short notation of the definition of logarithm:
if \(a^(b)=c\), then \(\log_(a)(c)=b\)
That is, \(b\) is the same as \(\log_(a)(c)\). Then we can write \(\log_(a)(c)\) instead of \(b\) in the formula \(a^(b)=c\). It turned out \(a^(\log_(a)(c))=c\) - the main logarithmic identity.
You can find other properties of logarithms. With their help, you can simplify and calculate the values of expressions with logarithms, which are difficult to calculate directly.
Example : Find the value of the expression \(36^(\log_(6)(5))\)
Solution :
Answer : \(25\)
How to write a number as a logarithm?
As mentioned above, any logarithm is just a number. The converse is also true: any number can be written as a logarithm. For example, we know that \(\log_(2)(4)\) is equal to two. Then instead of two you can write \(\log_(2)(4)\).
But \(\log_(3)(9)\) is also equal to \(2\), which means we can also write \(2=\log_(3)(9)\) . Likewise with \(\log_(5)(25)\), and with \(\log_(9)(81)\), etc. That is, it turns out
\(2=\log_(2)(4)=\log_(3)(9)=\log_(4)(16)=\log_(5)(25)=\log_(6)(36)=\ log_(7)(49)...\)
Thus, if we need, we can write two as a logarithm with any base anywhere (even in an equation, even in an expression, even in an inequality) - we simply write the squared base as an argument.
It’s the same with the triple – it can be written as \(\log_(2)(8)\), or as \(\log_(3)(27)\), or as \(\log_(4)(64) \)... Here we write the base in the cube as an argument:
\(3=\log_(2)(8)=\log_(3)(27)=\log_(4)(64)=\log_(5)(125)=\log_(6)(216)=\ log_(7)(343)...\)
And with four:
\(4=\log_(2)(16)=\log_(3)(81)=\log_(4)(256)=\log_(5)(625)=\log_(6)(1296)=\ log_(7)(2401)...\)
And with minus one:
\(-1=\) \(\log_(2)\)\(\frac(1)(2)\) \(=\) \(\log_(3)\)\(\frac(1)( 3)\) \(=\) \(\log_(4)\)\(\frac(1)(4)\) \(=\) \(\log_(5)\)\(\frac(1 )(5)\) \(=\) \(\log_(6)\)\(\frac(1)(6)\) \(=\) \(\log_(7)\)\(\frac (1)(7)\) \(...\)
And with one third:
\(\frac(1)(3)\) \(=\log_(2)(\sqrt(2))=\log_(3)(\sqrt(3))=\log_(4)(\sqrt( 4))=\log_(5)(\sqrt(5))=\log_(6)(\sqrt(6))=\log_(7)(\sqrt(7))...\)
Any number \(a\) can be represented as a logarithm with base \(b\): \(a=\log_(b)(b^(a))\)
Example : Find the meaning of the expression \(\frac(\log_(2)(14))(1+\log_(2)(7))\)
Solution :
Answer : \(1\)
Definition of logarithm
The logarithm of b to base a is the exponent to which a must be raised to get b.
Number e in mathematics it is customary to denote the limit to which an expression strives
Number e is irrational number- a number incommensurable with one, it cannot be accurately expressed as either an integer or a fraction rational number.
Letter e- the first letter of the Latin word exponere- to show off, hence the name in mathematics exponential- exponential function.
Number e widely used in mathematics, and in all sciences that in one way or another use mathematical calculations for their needs.
Logarithms. Properties of logarithms
Definition: The logarithm of a positive number b to its base is the exponent of c to which the number a must be raised to obtain the number b.
Basic logarithmic identity:
7) Formula for moving to a new base:
lna = log e a, e ≈ 2.718…
Problems and tests on the topic “Logarithms. Properties of logarithms"
- Logarithms - Important topics for reviewing the Unified State Examination in mathematics
To successfully complete tasks on this topic, you must know the definition of a logarithm, the properties of logarithms, the basic logarithmic identity, the definitions of decimal and natural logarithms. The main types of problems on this topic are problems involving the calculation and transformation of logarithmic expressions. Let's consider their solution using the following examples.
Solution: Using the properties of logarithms, we get
Solution: Using the properties of degrees, we get
1) (2 2) log 2 5 =(2 log 2 5) 2 =5 2 =25
Properties of logarithms, formulations and proofs.
Logarithms have a number of characteristic properties. In this article we will look at the main properties of logarithms. Here we will give their formulations, write the properties of logarithms in the form of formulas, show examples of their application, and also provide proof of the properties of logarithms.
Page navigation.
Basic properties of logarithms, formulas
For ease of remembering and use, let's imagine basic properties of logarithms in the form of a list of formulas. In the next paragraph we will give their formulations, evidence, examples of use and necessary explanations.
and the property of the logarithm of the product of n positive numbers: log a (x 1 · x 2 ·…·x n)= log a x 1 +log a x 2 +…+log a x n , a>0 , a≠1 , x 1 >0, x 2 >0, …, x n >0 .
, where a>0, a≠1, x>0, y>0.
, where a>0, a≠1, n is a natural number greater than one, b>0.
, a>0 , a≠1 , b>0 , b≠1 .
, a>0 , a≠1 , b>0 , p and q are real numbers, q≠0 , in particular for b=a we have 
.Formulations and proofs of properties
We proceed to the formulation and proof of the written properties of logarithms. All properties of logarithms are proven on the basis of the definition of the logarithm and the basic logarithmic identity that follows from it, as well as the properties of the degree.
Let's start with properties of the logarithm of one. Its formulation is as follows: the logarithm of unity is equal to zero, that is, log a 1=0 for any a>0, a≠1. The proof is not difficult: since a 0 =1 for any a satisfying the above conditions a>0 and a≠1, then the equality log a 1=0 to be proved follows immediately from the definition of the logarithm.
Let us give examples of the application of the considered property: log 3 1=0, log1=0 and .
Let's move on to the next property: the logarithm of a number equal to the base is equal to one, that is, log a a=1 for a>0, a≠1. Indeed, since a 1 =a for any a, then by definition of the logarithm log a a=1.
Examples of the use of this property of logarithms are the equalities log 5 5=1, log 5.6 5.6 and lne=1.
The logarithm of a power of a number equal to the base of the logarithm is equal to the exponent. This property of the logarithm corresponds to a formula of the form log a a p =p, where a>0, a≠1 and p – any real number. This property follows directly from the definition of the logarithm. Note that it allows you to immediately indicate the value of the logarithm, if it is possible to represent the number under the logarithm sign as a power of the base; we will talk more about this in the article calculating logarithms.
For example, log 2 2 7 =7, log10 -4 =-4 and 
.
Logarithm of the product of two positive numbers x and y is equal to the product of the logarithms of these numbers: log a (x y)=log a x+log a y, a>0 , a≠1 . Let us prove the property of the logarithm of a product. Due to the properties of the degree a log a x+log a y =a log a x ·a log a y, and since by the main logarithmic identity a log a x =x and a log a y =y, then a log a x ·a log a y =x· y. Thus, a log a x+log a y =x·y, from which, by the definition of a logarithm, the equality being proved follows.
Let's show examples of using the property of the logarithm of a product: log 5 (2 3)=log 5 2+log 5 3 and 
.
The property of the logarithm of a product can be generalized to the product of a finite number n of positive numbers x 1 , x 2 , …, x n as log a (x 1 · x 2 ·…·x n)= log a x 1 +log a x 2 +…+log a x n. This equality can be proven without problems using the method of mathematical induction.
For example, the natural logarithm of the product can be replaced by the sum of three natural logarithms of the numbers 4, e, and.
Logarithm of the quotient of two positive numbers x and y is equal to the difference between the logarithms of these numbers. The property of the logarithm of a quotient corresponds to a formula of the form , where a>0, a≠1, x and y are some positive numbers. The validity of this formula is proven as well as the formula for the logarithm of a product: since 
, then by definition of the logarithm .
Here is an example of using this property of the logarithm: 
.
Let's move on to property of the logarithm of the power. The logarithm of a degree is equal to the product of the exponent and the logarithm of the modulus of the base of this degree. Let us write this property of the logarithm of a power as a formula: log a b p =p·log a |b|, where a>0, a≠1, b and p are numbers such that the degree b p makes sense and b p >0.
First we prove this property for positive b. The basic logarithmic identity allows us to represent the number b as a log a b , then b p =(a log a b) p , and the resulting expression, due to the property of power, is equal to a p·log a b . So we come to the equality b p =a p·log a b, from which, by the definition of a logarithm, we conclude that log a b p =p·log a b.
It remains to prove this property for negative b. Here we note that the expression log a b p for negative b makes sense only for even exponents p (since the value of the degree b p must be greater than zero, otherwise the logarithm will not make sense), and in this case b p =|b| p. Then b p =|b| p =(a log a |b|) p =a p·log a |b| , from where log a b p =p·log a |b| .
For example, 
and ln(-3) 4 =4·ln|-3|=4·ln3 .
It follows from the previous property property of the logarithm from the root: the logarithm of the nth root is equal to the product of the fraction 1/n by the logarithm of the radical expression, that is, where a>0, a≠1, n is a natural number greater than one, b>0.
The proof is based on the equality (see definition of exponent with a fractional exponent), which is valid for any positive b, and the property of the logarithm of the exponent: 
.
Here is an example of using this property: 
.
Now let's prove formula for moving to a new logarithm base kind 
. To do this, it is enough to prove the validity of the equality log c b=log a b·log c a. The basic logarithmic identity allows us to represent the number b as a log a b , then log c b=log c a log a b . It remains to use the property of the logarithm of the degree: log c a log a b =log a b·log c a . This proves the equality log c b=log a b·log c a , which means the formula for transition to a new base of the logarithm is also proven .
Let's show a couple of examples of using this property of logarithms: and 
.
The formula for moving to a new base allows you to move on to working with logarithms that have a “convenient” base. For example, it can be used to change to natural or decimal logarithms so that you can calculate the value of a logarithm from a table of logarithms. The formula for moving to a new logarithm base also allows, in some cases, to find the value of a given logarithm when the values of some logarithms with other bases are known.
A special case of the formula for transition to a new logarithm base for c=b of the form is often used. This shows that log a b and log b a are mutually inverse numbers. For example, 
.
The formula is also often used, which is convenient for finding the values of logarithms. To confirm our words, we will show how it can be used to calculate the value of a logarithm of the form . We have 
. To prove the formula, it is enough to use the formula for moving to a new base of the logarithm a: 
.
It remains to prove the properties of comparison of logarithms.
Let's use the opposite method. Suppose that for a 1 >1, a 2 >1 and a 1 2 and for 0 1, log a 1 b≤log a 2 b is true. Based on the properties of logarithms, these inequalities can be rewritten as 
And 
respectively, and from them it follows that log b a 1 ≤log b a 2 and log b a 1 ≥log b a 2, respectively. Then, according to the properties of powers with the same bases, the equalities b log b a 1 ≥b log b a 2 and b log b a 1 ≥b log b a 2 must hold, that is, a 1 ≥a 2 . So we came to a contradiction to the condition a 1 2. This completes the proof.
Basic properties of logarithms
- Materials for the lesson
- Download all formulas
- log a x n = n · log a x ;
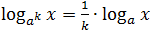
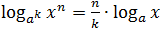
Logarithms, like any numbers, can be added, subtracted and transformed in every way. But since logarithms are not exactly ordinary numbers, there are rules here, which are called main properties.
You definitely need to know these rules - without them not a single serious logarithmic problem can be solved. In addition, there are very few of them - you can learn everything in one day. So let's get started.
Adding and subtracting logarithms
Consider two logarithms with the same bases: log a x and log a y. Then they can be added and subtracted, and:
So, the sum of logarithms is equal to the logarithm of the product, and the difference is equal to the logarithm of the quotient. Please note: the key point here is identical grounds. If the reasons are different, these rules do not work!
These formulas will help you calculate a logarithmic expression even when its individual parts are not considered (see the lesson “What is a logarithm”). Take a look at the examples and see:
Task. Find the value of the expression: log 6 4 + log 6 9.
Since logarithms have the same bases, we use the sum formula:
log 6 4 + log 6 9 = log 6 (4 9) = log 6 36 = 2.
Task. Find the value of the expression: log 2 48 − log 2 3.
The bases are the same, we use the difference formula:
log 2 48 − log 2 3 = log 2 (48: 3) = log 2 16 = 4.
Task. Find the value of the expression: log 3 135 − log 3 5.
Again the bases are the same, so we have:
log 3 135 − log 3 5 = log 3 (135: 5) = log 3 27 = 3.
As you can see, the original expressions are made up of “bad” logarithms, which are not calculated separately. But after the transformations, completely normal numbers are obtained. Many tests are based on this fact. Yes, test-like expressions are offered in all seriousness (sometimes with virtually no changes) on the Unified State Examination.
Extracting the exponent from the logarithm
Now let's complicate the task a little. What if the base or argument of a logarithm is a power? Then the exponent of this degree can be taken out of the sign of the logarithm according to the following rules:
It is easy to see that the last rule follows the first two. But it’s better to remember it anyway - in some cases it will significantly reduce the amount of calculations.
Of course, all these rules make sense if the ODZ of the logarithm is observed: a > 0, a ≠ 1, x > 0. And one more thing: learn to apply all formulas not only from left to right, but also vice versa, i.e. You can enter the numbers before the logarithm sign into the logarithm itself. This is what is most often required.
Task. Find the value of the expression: log 7 49 6 .
Let's get rid of the degree in the argument using the first formula:
log 7 49 6 = 6 log 7 49 = 6 2 = 12
Task. Find the meaning of the expression:
[Caption for the picture]
Note that the denominator contains a logarithm, the base and argument of which are exact powers: 16 = 2 4 ; 49 = 7 2. We have:
[Caption for the picture]
I think the last example requires some clarification. Where have logarithms gone? Until the very last moment we work only with the denominator. We presented the base and argument of the logarithm standing there in the form of powers and took out the exponents - we got a “three-story” fraction.
Now let's look at the main fraction. The numerator and denominator contain the same number: log 2 7. Since log 2 7 ≠ 0, we can reduce the fraction - 2/4 will remain in the denominator. According to the rules of arithmetic, the four can be transferred to the numerator, which is what was done. The result was the answer: 2.
Transition to a new foundation
Speaking about the rules for adding and subtracting logarithms, I specifically emphasized that they only work with the same bases. What if the reasons are different? What if they are not exact powers of the same number?
Formulas for transition to a new foundation come to the rescue. Let us formulate them in the form of a theorem:
Let the logarithm log a x be given. Then for any number c such that c > 0 and c ≠ 1, the equality is true:
[Caption for the picture]
In particular, if we set c = x, we get:
[Caption for the picture]
From the second formula it follows that the base and argument of the logarithm can be swapped, but in this case the entire expression is “turned over”, i.e. the logarithm appears in the denominator.
These formulas are rarely found in ordinary numerical expressions. It is possible to evaluate how convenient they are only when solving logarithmic equations and inequalities.
However, there are problems that cannot be solved at all except by moving to a new foundation. Let's look at a couple of these:
Task. Find the value of the expression: log 5 16 log 2 25.
Note that the arguments of both logarithms contain exact powers. Let's take out the indicators: log 5 16 = log 5 2 4 = 4log 5 2; log 2 25 = log 2 5 2 = 2log 2 5;
Now let’s “reverse” the second logarithm:
[Caption for the picture]
Since the product does not change when rearranging factors, we calmly multiplied four and two, and then dealt with logarithms.
Task. Find the value of the expression: log 9 100 lg 3.
The base and argument of the first logarithm are exact powers. Let's write this down and get rid of the indicators:
[Caption for the picture]
Now let's get rid of the decimal logarithm by moving to a new base:
[Caption for the picture]
Basic logarithmic identity
Often in the solution process it is necessary to represent a number as a logarithm to a given base. In this case, the following formulas will help us:
- n = log a a n
-
In the first case, the number n becomes the exponent in the argument. The number n can be absolutely anything, because it is just a logarithm value.
The second formula is actually a paraphrased definition. That’s what it’s called: the basic logarithmic identity.
In fact, what happens if the number b is raised to such a power that the number b to this power gives the number a? That's right: the result is the same number a. Read this paragraph carefully again - many people get stuck on it.
Like formulas for moving to a new base, the basic logarithmic identity is sometimes the only possible solution.
[Caption for the picture]
Note that log 25 64 = log 5 8 - we simply took the square from the base and argument of the logarithm. Taking into account the rules for multiplying powers with the same base, we get:
[Caption for the picture]
If anyone doesn’t know, this was a real task from the Unified State Exam :)
Logarithmic unit and logarithmic zero
In conclusion, I will give two identities that can hardly be called properties - rather, they are consequences of the definition of the logarithm. They constantly appear in problems and, surprisingly, create problems even for “advanced” students.
- log a a = 1 is a logarithmic unit. Remember once and for all: the logarithm to any base a of that base itself is equal to one.
- log a 1 = 0 is logarithmic zero. The base a can be anything, but if the argument contains one, the logarithm is equal to zero! Because a 0 = 1 is a direct consequence of the definition.
That's all the properties. Be sure to practice putting them into practice! Download the cheat sheet at the beginning of the lesson, print it out, and solve the problems.
Logarithm. Properties of the logarithm (addition and subtraction).
Properties of the logarithm follow from its definition. And so the logarithm of the number b based on A is defined as the exponent to which a number must be raised a to get the number b(logarithm exists only for positive numbers).
From this formulation it follows that the calculation x=log a b, is equivalent to solving the equation a x =b. For example, log 2 8 = 3 because 8 = 2 3 . The formulation of the logarithm makes it possible to justify that if b=a c, then the logarithm of the number b based on a equals With. It is also clear that the topic of logarithms is closely related to the topic of powers.
With logarithms, as with any numbers, you can do operations of addition, subtraction and transform in every possible way. But due to the fact that logarithms are not entirely ordinary numbers, their own special rules apply here, which are called main properties.
Adding and subtracting logarithms.
Let's take two logarithms with the same bases: log a x And log a y. Then it is possible to perform addition and subtraction operations:
As we see, sum of logarithms equals the logarithm of the product, and difference logarithms- logarithm of the quotient. Moreover, this is true if the numbers A, X And at positive and a ≠ 1.
It is important to note that the main aspect in these formulas are the same bases. If the grounds are different, these rules do not apply!
The rules for adding and subtracting logarithms with the same bases are read not only from left to right, but also vice versa. As a result, we have the theorems for the logarithm of the product and the logarithm of the quotient.
Logarithm of the product two positive numbers equal to the sum their logarithms ; paraphrasing this theorem we get the following if the numbers A, x And at positive and a ≠ 1, That:
Logarithm of the quotient two positive numbers is equal to the difference between the logarithms of the dividend and the divisor. To put it another way, if the numbers A, X And at positive and a ≠ 1, That:
Let us apply the above theorems to solve examples:
If the numbers x And at are negative, then product logarithm formula becomes meaningless. Thus, it is forbidden to write:
since the expressions log 2 (-8) and log 2 (-4) are not defined at all (logarithmic function at= log 2 X defined only for positive argument values X).
Product theorem applicable not only for two, but also for an unlimited number of factors. This means that for every natural k and any positive numbers x 1 , x 2 , . . . ,x n there is an identity:
From logarithm quotient theorem one more property of the logarithm can be obtained. It is common knowledge that log a 1= 0, therefore
This means there is an equality:
Logarithms of two reciprocal numbers for the same reason will differ from each other solely by sign. So:
Logarithm. Properties of logarithms
Logarithm. Properties of logarithms
Let's consider equality. Let us know the values of and and we want to find the value of .
That is, we are looking for the exponent by which we need to cock it to get .
Let
a variable can take on any real value, then the following restrictions are imposed on the variables: o" title=»a>o»/> , 1″ title=»a1″/>, 0″ title=»b>0″/>If we know the values of and , and we are faced with the task of finding the unknown, then for this purpose a mathematical operation is introduced, which is called logarithm.
To find the value we take logarithm of a number By basis :
The logarithm of a number to its base is the exponent to which it must be raised to get .
That is basic logarithmic identity:
o» title=»a>o»/> , 1″ title=»a1″/>, 0″ title=»b>0″/>
is essentially a mathematical notation definitions of logarithm.
The mathematical operation of logarithm is the inverse of the operation of exponentiation, so properties of logarithms are closely related to the properties of degree.
Let's list the main properties of logarithms:
(o" title="a>o"/> , 1″ title=»a1″/>, 0″ title=»b>0″/>, 0,
d>0″/>, 1″ title=”d1″/>
4.
5.
The following group of properties allows you to represent the exponent of an expression under the sign of the logarithm, or standing at the base of the logarithm in the form of a coefficient in front of the sign of the logarithm:
6.
7.
8.
9.
The next group of formulas allows you to move from a logarithm with a given base to a logarithm with an arbitrary base, and is called formulas for moving to a new base:
10.
12. (corollary from property 11)
The following three properties are not well known, but they are often used when solving logarithmic equations, or when simplifying expressions containing logarithms:
13.
14.
15.
Special cases:
— decimal logarithm
— natural logarithmWhen simplifying expressions containing logarithms, a general approach is used:
1. Introducing decimals in the form of ordinary ones.
2. Mixed numbers represented as improper fractions.
3. We decompose the numbers at the base of the logarithm and under the sign of the logarithm into simple factors.
4. We try to reduce all logarithms to the same base.
5. Apply the properties of logarithms.
Let's look at examples of simplifying expressions containing logarithms.
Example 1.
Calculate:
Let's simplify all exponents: our task is to reduce them to logarithms, the base of which is the same number as the base of the exponent.
==(by property 7)=(by property 6) =
Let's substitute the indicators that we got into the original expression. We get:
Answer: 5.25
Example 2. Calculate:
Let's reduce all logarithms to base 6 (in this case, the logarithms from the denominator of the fraction will “migrate” to the numerator):
Let's decompose the numbers under the logarithm sign into simple factors:
Let's apply properties 4 and 6:
Let's introduce the replacement
We get:
Answer: 1
Logarithm . Basic logarithmic identity.
Properties of logarithms. Decimal logarithm. Natural logarithm.
Logarithm positive number N to base (b > 0, b 1) is the exponent x to which b must be raised to get N .
This entry is equivalent to the following: b x = N .
Examples: log 3 81 = 4, since 3 4 = 81;
log 1/3 27 = – 3, since (1/3) - 3 = 3 3 = 27.
The above definition of logarithm can be written as an identity:
Basic properties of logarithms.
2) log 1 = 0, since b 0 = 1 .
3) The logarithm of the product is equal to the sum of the logarithms of the factors:
4) The logarithm of the quotient is equal to the difference between the logarithms of the dividend and the divisor:
5) The logarithm of a power is equal to the product of the exponent and the logarithm of its base:
The consequence of this property is the following: logarithm of the root equal to the logarithm of the radical number divided by the power of the root:
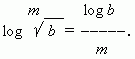
6) If the base of the logarithm is a degree, then the value the inverse of the exponent can be taken out as a log rhyme:
The last two properties can be combined into one:
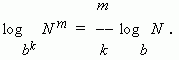
7) Transition modulus formula (i.e. transition from one logarithm base to another base):
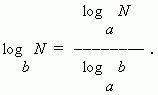
In the special case when N=a we have:
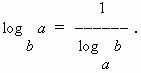
Decimal logarithm called base logarithm 10. It is denoted lg, i.e. log 10 N= log N. Logarithms of numbers 10, 100, 1000, . p are 1, 2, 3, …, respectively, i.e. have so many positive
units, how many zeros are there in a logarithmic number after one. Logarithms of numbers 0.1, 0.01, 0.001, . p are respectively –1, –2, –3, …, i.e. have as many negative ones as there are zeros in the logarithmic number before one (including zero integers). The logarithms of other numbers have a fractional part called mantissa. The integer part of a logarithm is called characteristic. For practical use, decimal logarithms are most convenient.
Natural logarithm called base logarithm e. It is denoted by ln, i.e. log e N= log N. Number e is irrational, its approximate value is 2.718281828. It is the limit to which the number tends (1 + 1 / n) n with unlimited increase n(cm. first wonderful limit on the "Limits" page number sequences»).
Strange as it may seem, natural logarithms turned out to be very convenient when carrying out various types of operations related to the analysis of functions. Calculating logarithms to the base e carried out much faster than for any other reason.
- What is needed today to adopt a child in Russia? Adoption in Russia, in addition to a responsible personal decision, involves a number of procedures for state verification of candidates. Rigorous selection at the preparatory stage contributes to more […]
- Free information on TIN or OGRN from the tax register throughout Russia - online On the Unified Tax Services Portal you can obtain information about state registration legal entities, individual entrepreneurs, […]
- Punishment for driving without documents (driver's license, insurance, STS) Sometimes, due to forgetfulness, drivers get behind the wheel without a license and receive a fine for driving without documents. We would like to remind you that a car enthusiast is required to have […]
- Flowers for men. What flowers can you give a man? What flowers can you give a man? There are not many “male” flowers, but there are some that are given to men. A small flower list in front of you: Chrysanthemums. Roses. Carnations. […]
- An internal memo is a special form of document that is used in the internal environment of an enterprise and serves to quickly resolve current production problems. Typically this document is drawn up for the purpose of introducing some […]
- When and how to receive the funded part of your pension from Sberbank? Sberbank is a partner bank of the state pension fund. Based on this, citizens who registered for a funded pension could transfer the funded part […]
- Child benefits in Ulyanovsk and the Ulyanovsk region in 2018 In addition, programs approved by federal legislation operate in all regions. Let's look at who can count on what benefits. How regional authorities […]
- Detailed Guide how to draw up a power of attorney to represent interests individual in court In a civil or arbitration claim, in an administrative or criminal case, the interests of both the plaintiff and the defendant can be represented by an attorney: […]