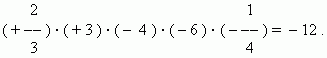>

How to find the sum of numbers with different signs. Adding numbers with different signs, rules, examples

>>Math: Adding numbers with different signs

33. Addition of numbers with different signs

If the air temperature was equal to 9 °C, and then it changed to - 6 °C (i.e., decreased by 6 °C), then it became equal to 9 + (- 6) degrees (Fig. 83).

To add the numbers 9 and - 6 using , you need to move point A (9) to the left by 6 unit segments (Fig. 84). We get point B (3).

This means 9+(- 6) = 3. The number 3 has the same sign as the term 9, and its module equal to the difference between the moduli of terms 9 and -6.

Indeed, |3| =3 and |9| - |- 6| = = 9 - 6 = 3.

If the same air temperature of 9 °C changed by -12 °C (i.e., decreased by 12 °C), then it became equal to 9 + (-12) degrees (Fig. 85). Adding the numbers 9 and -12 using the coordinate line (Fig. 86), we get 9 + (-12) = -3. The number -3 has the same sign as the term -12, and its module is equal to the difference between the modules of the terms -12 and 9.

Indeed, | - 3| = 3 and | -12| - | -9| =12 - 9 = 3.

To add two numbers with different signs, you need to:

1) subtract the smaller one from the larger module of the terms;

2) put in front of the resulting number the sign of the term whose modulus is greater.

Usually, the sign of the sum is first determined and written, and then the difference in modules is found.

For example:

1) 6,1+(- 4,2)= +(6,1 - 4,2)= 1,9,
or shorter 6.1+(- 4.2) = 6.1 - 4.2 = 1.9;

When adding positive and negative numbers you can use micro calculator. To enter a negative number into a microcalculator, you need to enter the modulus of this number, then press the “change sign” key |/-/|. For example, to enter the number -56.81, you must press the keys sequentially: | 5 |, | 6 |, | ¦ |, | 8 |, | 1 |, |/-/|. Operations on numbers of any sign are performed on a microcalculator in the same way as on positive numbers.

For example, the sum -6.1 + 3.8 is calculated by program

? The numbers a and b have different signs. What sign will the sum of these numbers have if the larger module is negative?

if the smaller modulus is negative?

if the larger modulus is a positive number?

if the smaller modulus is a positive number?

Formulate a rule for adding numbers with different signs. How to enter a negative number into a microcalculator?

TO 1045. The number 6 was changed to -10. On which side of the origin is the resulting number located? At what distance from the origin is it located? What is it equal to sum 6 and -10?

1046. The number 10 was changed to -6. On which side of the origin is the resulting number located? At what distance from the origin is it located? What is the sum of 10 and -6?

1047. The number -10 was changed to 3. On which side of the origin is the resulting number located? At what distance from the origin is it located? What is the sum of -10 and 3?

1048. The number -10 was changed to 15. On which side of the origin is the resulting number located? At what distance from the origin is it located? What is the sum of -10 and 15?

1049. In the first half of the day the temperature changed by - 4 °C, and in the second half - by + 12 °C. By how many degrees did the temperature change during the day?

1050. Perform addition:

1051. Add:

a) to the sum of -6 and -12 the number 20;
b) to the number 2.6 the sum is -1.8 and 5.2;
c) to the sum -10 and -1.3 the sum of 5 and 8.7;
d) to the sum of 11 and -6.5 the sum of -3.2 and -6.

1052. Which number is 8; 7.1; -7.1; -7; -0.5 is the root equations- 6 + x = -13.1?

1053. Guess the root of the equation and check:

a) x + (-3) = -11; c) m + (-12) = 2;
b) - 5 + y=15; d) 3 + n = -10.

1054. Find the meaning of the expression:

1055. Follow the steps using a microcalculator:

a) - 3.2579 + (-12.308); d) -3.8564+ (-0.8397) +7.84;
b) 7.8547+ (- 9.239); e) -0.083 + (-6.378) + 3.9834;
c) -0.00154 + 0.0837; e) -0.0085+ 0.00354+ (- 0.00921).

P 1056. Find the value of the sum:

1057. Find the meaning of the expression:

1058. How many integers are located between the numbers:

a) 0 and 24; b) -12 and -3; c) -20 and 7?

1059. Imagine the number -10 as the sum of two negative terms so that:

a) both terms were integers;
b) both terms were decimal fractions;
c) one of the terms was a regular ordinary fraction.

1060. What is the distance (in unit segments) between the points of the coordinate line with coordinates:

a) 0 and a; b) -a and a; c) -a and 0; d) a and -Za?

M 1061. Radii of geographical parallels earth's surface, on which the cities of Athens and Moscow are located, are respectively 5040 km and 3580 km (Fig. 87). How much shorter is the Moscow parallel than the Athens parallel?

1062. Write an equation to solve the problem: “A field with an area of ​​2.4 hectares was divided into two sections. Find square each site, if it is known that one of the sites:

a) 0.8 hectares more than another;
b) 0.2 hectares less than another;
c) 3 times more than another;
d) 1.5 times less than another;
e) constitutes another;
e) is 0.2 of the other;
g) constitutes 60% of the other;
h) is 140% of the other.”

1063. Solve the problem:

1) On the first day, the travelers traveled 240 km, on the second day 140 km, on the third day they traveled 3 times more than on the second, and on the fourth day they rested. How many kilometers did they travel on the fifth day, if over 5 days they drove an average of 230 km per day?

2) Father’s monthly income is 280 rubles. My daughter's scholarship is 4 times less. How much does a mother earn per month if there are 4 people in the family? younger son- a schoolboy and each person receives an average of 135 rubles?

1064. Follow these steps:

1) (2,35 + 4,65) 5,3:(40-2,9);

2) (7,63-5,13) 0,4:(3,17 + 6,83).

1066. Present each of the numbers as a sum of two equal terms:

1067. Find the value of a + b if:

a) a= -1.6, b = 3.2; b) a=- 2.6, b = 1.9; V)

1068. There were 8 apartments on one floor of a residential building. 2 apartments had a living area of ​​22.8 m2, 3 apartments - 16.2 m2, 2 apartments - 34 m2. What living area did the eighth apartment have if on this floor on average each apartment had 24.7 m2 of living space?

1069. The freight train consisted of 42 cars. There were 1.2 times more covered cars than platforms, and the number of tanks was equal to the number of platforms. How many cars of each type were on the train?

1070. Find the meaning of the expression

N.Ya.Vilenkin, A.S. Chesnokov, S.I. Shvartsburd, V.I. Zhokhov, Mathematics for grade 6, Textbook for high school

Mathematics planning, textbooks and books online, courses and tasks in mathematics for 6th grade download

Lesson content lesson notes supporting frame lesson presentation acceleration methods interactive technologies Practice tasks and exercises self-test workshops, trainings, cases, quests homework discussion questions rhetorical questions from students Illustrations audio, video clips and multimedia photographs, pictures, graphics, tables, diagrams, humor, anecdotes, jokes, comics, parables, sayings, crosswords, quotes Add-ons abstracts articles tricks for the curious cribs textbooks basic and additional dictionary of terms other Improving textbooks and lessonscorrecting errors in the textbook updating a fragment in a textbook, elements of innovation in the lesson, replacing outdated knowledge with new ones Only for teachers perfect lessons calendar plan for a year guidelines discussion programs Integrated Lessons

Addition of negative numbers.

The sum of negative numbers is a negative number. Sum module equal to the sum modules of terms.

Let's figure out why the sum of negative numbers will also be a negative number. The coordinate line will help us with this, on which we will add the numbers -3 and -5. Let us mark a point on the coordinate line corresponding to the number -3.

To the number -3 we need to add the number -5. Where do we go from the point corresponding to the number -3? That's right, left! For 5 unit segments. We mark a point and write the number corresponding to it. This number is -8.

So, when adding negative numbers using the coordinate line, we are always to the left of the origin, therefore, it is clear that the result of adding negative numbers is also a negative number.

Note. We added the numbers -3 and -5, i.e. found the value of the expression -3+(-5). Usually, when adding rational numbers, they simply write down these numbers with their signs, as if listing all the numbers that need to be added. This notation is called an algebraic sum. Apply (in our example) the entry: -3-5=-8.

Example. Find the sum of negative numbers: -23-42-54. (Do you agree that this entry is shorter and more convenient like this: -23+(-42)+(-54))?

Let's decide according to the rule for adding negative numbers: we add the modules of the terms: 23+42+54=119. The result will have a minus sign.

They usually write it like this: -23-42-54=-119.

Addition of numbers with different signs.

The sum of two numbers with different signs has the sign of a term with a large absolute value. To find the modulus of a sum, you need to subtract the smaller modulus from the larger modulus..

Let's perform the addition of numbers with different signs using a coordinate line.

1) -4+6. You need to add the number 6 to the number -4. Let's mark the number -4 with a dot on the coordinate line. The number 6 is positive, which means that from the point with coordinate -4 we need to go to the right by 6 unit segments. We found ourselves to the right of the reference point (from zero) by 2 unit segments.

The result of the sum of the numbers -4 and 6 is the positive number 2:

- 4+6=2. How could you get the number 2? Subtract 4 from 6, i.e. subtract the smaller one from the larger module. The result has the same sign as the term with a large modulus.

2) Let's calculate: -7+3 using the coordinate line. Mark the point corresponding to the number -7. We go to the right for 3 unit segments and get a point with coordinate -4. We were and remain to the left of the origin: the answer is a negative number.

— 7+3=-4. We could get this result this way: from the larger module we subtracted the smaller one, i.e. 7-3=4. As a result, we put the sign of the term with the larger modulus: |-7|>|3|.

Examples. Calculate: A) -4+5-9+2-6-3; b) -10-20+15-25.

“Adding numbers with different signs” - Mathematics textbook, grade 6 (Vilenkin)

Short description:


In this section you will learn the rules for adding numbers with different signs: that is, you will learn to add negative and positive numbers.
You already know how to add them on a coordinate line, but in each example you won’t draw a line and count using it? Therefore, you need to learn how to fold without it.
Let's try with you to add a negative number to a positive number, for example eight add minus six: 8+(-6). You already know that adding a negative number reduces the original number by a negative value. This means that eight must be reduced by six, that is, six must be subtracted from eight: 8-6 = 2, which gives two. In this example, everything seems to be clear; we subtract six from eight.
And if we take this example: add a positive number to a negative number. For example, minus eight add six: -8+6. The essence remains the same: we reduce a positive number by the value of a negative one, we get six subtract eight is minus two: -8+6=-2.
As you noticed, in both the first and second examples with numbers, the action of subtraction is performed. Why? Because they have different signs (plus and minus). To avoid making mistakes when adding numbers with different signs, you should perform the following algorithm:
1. find the modules of numbers;
2. subtract the smaller module from the larger module;
3. Before the result obtained, put a number sign with a large absolute value (usually only a minus sign is put, and a plus sign is not put).
If you add numbers with different signs following this algorithm, then you will have much less chance of making a mistake.

If the air temperature was 9°C, and then it changed to -6°C (i.e., decreased by 6°C), then it became equal to 9 + (-6) degrees (Fig. 83).

Rice. 83

To add the numbers 9 and -6 using the coordinate line, you need to move point A(9) to the left by 6 unit segments (Fig. 84). We get point B(3).

Rice. 84

This means 9 + (-6) = 3. The number 3 has the same sign as the term 9, and its module is equal to the difference between the modules of the terms 9 and -6.

Indeed, |3| = 3 and |9| - |-6| = 9 - 6 = 3.

If the same air temperature of 9°C changed by -12°C (i.e. decreased by 12°C), then it became equal to 9 + (-12) degrees (Fig. 85).

Rice. 85

Adding the numbers 9 and -12 using the coordinate line (Fig. 86), we get 9 + (-12) = -3. The number -3 has the same sign as the term -12, and its module is equal to the difference between the modules of the terms -12 and 9.

Rice. 86

Indeed, |-3| = 3 and |-12| - |-9| = 12 - 9 = 3.

Usually, the sign of the sum is first determined and written, and then the difference in modules is found.

For example:

You can use a calculator to add positive and negative numbers. To enter a negative number into a microcalculator, you need to enter the modulus of this number, then press the “change sign” key. For example, to enter the number -56.81, you need to press the keys sequentially: . Operations on numbers of any sign are performed on a microcalculator in the same way as on positive numbers. For example, the sum -6.1 + 3.8 is calculated using the program

In short, this program is written like this: .

Self-test questions

  • The numbers a and b have different signs. What sign will the sum of these numbers have if the larger module is negative? if the smaller modulus is negative? if the larger modulus is a positive number? if the smaller modulus is a positive number?
  • Formulate a rule for adding numbers with different signs.
  • How to enter a negative number into a microcalculator?

Do the exercises

1061. The number 6 was changed to -10. On which side of the origin is the resulting number located? At what distance from the origin is it located? What is the sum of 6 and -10?

1062. The number 10 was changed to -6. On which side of the origin is the resulting number located? At what distance from the origin is it located? What is the sum of 10 and -6?

1063. The number -10 was changed to 3. On which side of the origin is the resulting number located? At what distance from the origin is it located? What is the sum of -10 and 3?

1064. The number -10 was changed to 15. On which side of the origin is the resulting number located? At what distance from the origin is it located? What is the sum of -10 and 15?

1065. In the first half of the day the temperature changed by -4°C, and in the second - by +12°C. By how many degrees did the temperature change during the day?

1066. Perform addition:

  • a) 26 + (-6);
  • b) -70 + 50;
  • c) -17 + 30;
  • d) 80 + (-120);
  • e) -6.3 + 7.8;
  • e) -9 + 10.2;
  • g) 1 + (-0.39);
  • h) 0.3 + (-1.2);

1067. Add:

  • a) to the sum of -6 and -12 the number 20;
  • b) to the number 2.6 the sum is -1.8 and 5.2;
  • c) to the sum -10 and -1.3 the sum of 5 and 8.7;
  • d) to the sum of 11 and -6.5 the sum of -3.2 and -6.

1068. Which number is 8? 7.1; -7.1; -7; Is -0.5 the root of the equation -6 + x = -13.1?

1069. Guess the root of the equation and check:

  • a) x + (-3) = -11;
  • b) -5 + y = 15;
  • c) t + (-12) = 2;
  • d) 3 + n = -10.

1070. Find the meaning of the expression:

1071. Follow these steps using a microcalculator:

  • a) -3.2579 + (-12.308);
  • b) 7.8547 + (-9.239);
  • c) -0.00154 + 0.0837;
  • d) -3.8564 + (-0.8397) + 7.84;
  • e) -0.083 + (-6.378) + 3.9834;
  • e) -0.0085 + 0.00354 + (-0.00921).

1072. Find the value of the sum:

1073. Find the meaning of the expression:

1074. How many integers are located between the numbers:

  • a) 0 and 24;
  • b) -12 and -3;
  • c) -20 and 7?

1075. Imagine the number -10 as the sum of two negative terms so that:

  • a) both terms were integers;
  • b) both terms were decimal fractions;
  • c) one of the terms was a proper ordinary fraction.

1076. What is the distance (in unit segments) between points on a coordinate line with coordinates:

  • a) 0 and a;
  • b) -a and a;
  • c) -a and 0;
  • d) a and -Za?

1077. The radii of the geographical parallels of the earth's surface on which the cities of Athens and Moscow are located are respectively equal to 5040 km and 3580 km (Fig. 87). How much shorter is the Moscow parallel than the Athens parallel?

Rice. 87

1078. Write an equation to solve the problem: “A field of 2.4 hectares was divided into two sections. Find the area of ​​each plot if it is known that one of the plots:

1079. Solve the problem:

  1. On the first day, the travelers traveled 240 km, on the second day 140 km, on the third day they traveled 3 times more than on the second, and on the fourth day they rested. How many kilometers did they travel on the fifth day, if over 5 days they drove an average of 230 km per day?
  2. A farmer with two sons placed the collected apples in 4 containers, on average 135 kg each. The farmer collected 280 kg of apples, and the youngest son collected 4 times less. How many kilograms of apples did the eldest son collect?

1080. Follow these steps:

  1. (2,35 + 4,65) 5,3: (40 - 2,9);
  2. (7,63 - 5,13) 0,4: (3,17 + 6,83).

1081. Perform addition:

1082. Imagine each of the numbers as the sum of two equal terms: 10; -8; -6.8; .

1083. Find the value of a + b if:

1084. There were 8 apartments on one floor of a residential building. There were 2 apartments with a living area of ​​22.8 m2, 3 apartments with 16.2 m2, and 2 apartments with 34 m2. What living area did the eighth apartment have if on this floor on average each apartment had 24.7 m2 of living space?

1085. The freight train consisted of 42 cars. There were 1.2 times more covered cars than platforms, and the number of tanks was equal to the number of platforms. How many cars of each type were on the train?

1086. Find the meaning of the expression

In this article we will deal with adding numbers with different signs. Here we will give a rule for adding positive and negative numbers, and consider examples of the application of this rule when adding numbers with different signs.

Page navigation.

Rule for adding numbers with different signs

Positive and negative numbers can be interpreted as property and debt, respectively, while the modules of numbers show the amount of property and debt. Then the addition of numbers with different signs can be considered as the addition of property and debt. It is clear that if the property is less than the debt, then after offset there will be a debt, if the property is greater than the debt, then after offset there will be property, and if the property is equal to the debt, then after settlement there will be neither debt nor property.

Let us combine the above arguments into rule for adding numbers with different signs. To add a positive and negative number, you need to:

  • find the modules of the terms;
  • compare the numbers obtained, while
    • if the resulting numbers are equal, then the original terms are opposite numbers and their sum is zero,
    • if the resulting numbers are not equal, then you need to remember the sign of the number whose modulus is greater;
  • subtract the smaller one from the larger module;
  • Before the resulting number put the sign of the term whose modulus is greater.

    • The stated rule reduces the addition of numbers with different signs to the subtraction of a smaller number from a larger positive number. It is also clear that as a result of adding a positive and a negative number, you can get either a positive number, or a negative number, or zero.

    Also note that the rule for adding numbers with different signs is valid for integers, for rational numbers and for real numbers.

    Examples of adding numbers with different signs

    Let's consider examples of adding numbers with different signs according to the rule discussed in the previous paragraph. Let's start with a simple example.

    www.cleverstudents.ru

    Adding and subtracting fractions

    Fractions are ordinary numbers and can also be added and subtracted. But because they have a denominator, they require more complex rules than for integers.

    Let's consider the simplest case, when there are two fractions with the same denominators. Then:

    To add fractions with the same denominators, you need to add their numerators and leave the denominator unchanged.

    To subtract fractions with the same denominators, you need to subtract the numerator of the second from the numerator of the first fraction, and again leave the denominator unchanged.

    Task. Find the meaning of the expression:

    Within each expression, the denominators of the fractions are equal. By definition of adding and subtracting fractions we get:

    As you can see, nothing complicated: just add or subtract the numerators - that’s all.

    But even in such simple actions, people manage to make mistakes. What is most often forgotten is that the denominator does not change. For example, when adding them, they also begin to add up, and this is fundamentally wrong.

    Get rid of bad habit Adding the denominators is quite simple. Try the same thing when subtracting. As a result, the denominator will be zero, and the fraction will (suddenly!) lose its meaning.

    Therefore, remember once and for all: when adding and subtracting, the denominator does not change!

    Many people also make mistakes when adding several negative fractions. There is confusion with the signs: where to put a minus and where to put a plus.

    This problem is also very easy to solve. It is enough to remember that the minus before the sign of a fraction can always be transferred to the numerator - and vice versa. And of course, don’t forget two simple rules:

  • Plus by minus gives minus;
  • Two negatives make an affirmative.

    • Let's look at all this with specific examples:

    In the first case, everything is simple, but in the second, let’s add minuses to the numerators of the fractions:

    What to do if the denominators are different

    Directly adding fractions with different denominators it is forbidden. At least, this method is unknown to me. However, the original fractions can always be rewritten so that the denominators become the same.

    There are many ways to convert fractions. Three of them are discussed in the lesson “Reducing fractions to common denominator", so we will not dwell on them here. Let's look at some examples:

    In the first case, we reduce the fractions to a common denominator using the “criss-cross” method. In the second we will look for the NOC. Note that 6 = 2 · 3; 9 = 3 · 3. The last factors in these expansions are equal, and the first ones are relatively prime. Therefore, LCM(6, 9) = 2 3 3 = 18.

    What to do if a fraction has an integer part

    I can please you: different denominators in fractions are not the biggest evil. Much more errors occur when the whole part is highlighted in the addend fractions.

    Of course, there are own addition and subtraction algorithms for such fractions, but they are quite complex and require a long study. Better use simple diagram, given below:

  • Convert all fractions containing an integer part to improper ones. We obtain normal terms (even with different denominators), which are calculated according to the rules discussed above;
  • Actually, calculate the sum or difference of the resulting fractions. As a result, we will practically find the answer;
  • If this is all that was required in the problem, we perform the inverse transformation, i.e. We get rid of an improper fraction by highlighting the whole part.

    • The rules for moving to improper fractions and highlighting the whole part are described in detail in the lesson “What is a numerical fraction.” If you don’t remember, be sure to repeat it. Examples:

    Everything is simple here. The denominators inside each expression are equal, so all that remains is to convert all fractions to improper ones and count. We have:

    To simplify the calculations, I have skipped some obvious steps in the last examples.

    A small note about the last two examples, where fractions with the integer part highlighted are subtracted. The minus before the second fraction means that the entire fraction is subtracted, and not just its whole part.

    Re-read this sentence again, look at the examples - and think about it. This is where beginners admit great amount errors. They love to give such tasks to tests. You will also encounter them several times in the tests for this lesson, which will be published shortly.

    Summary: general calculation scheme

    In conclusion, I will give a general algorithm that will help you find the sum or difference of two or more fractions: